| Abstract: |
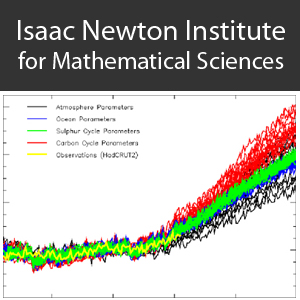
Recently, a conditional form of the Maximum Entropy Production (MaxEP) principle was derived based on Jaynes' maximum entropy method, using an entropy defined on the fluxes through an infinitesimal element of a flow system. The analysis is analogous to Gibbs' formulation of equilibrium thermodynamics, expressing the balance between changes in entropy inside and outside a system, but is formulated for a non-equilibrium flow system. The MaxEP principle emerges as the extremum condition in some circumstances. In this seminar, a generic form of the derivation is first provided, encompassing seemingly disparate formulations of equilibrium thermodynamics, local steady-state flow and global steady-state flow (and other systems). The implications of the analysis are explored for several systems, including (i) the transition between laminar and turbulent flow in a pipe, and (ii) the modelling of planetary climate systems, including solar and extrasolar planets. |